How imperative is it to skill our engineers? When does skilling really start? At the post-graduate level? Graduate? School?
Let me narrate a recent incident to you, and then you can draw your own conclusions.
This is how an engineering graduate with a further six months training in embedded systems, attempted to solve a simple exercise that I had given her:
The task was to calculate digital samples for generating a sinewave. I casually suggested that she could use Excel, if she wanted. She looked quite puzzled and asked ‘How can Excel calculate the samples’? I said, ‘Can’t you give a formula’? She asked, ‘What formula?’ I said, ‘If you specify ‘x’, the computer can calculate ‘sin x’. Anyway, I said she could do it manually with a calculator also if she so wished.
She came back to me with a table written on her notebook with columns of ‘x in 1 degree increments’, ‘x in radians’, ‘sin x in decimal’, ‘Hex value in 8 bits’. She had stopped at 15 degrees since it was taking her too much time to manually calculate the entire 360 degrees. I also noticed that she had not taken the negative values of sin x. So, I asked her to calculate just one sample in the 2nd and 3rd quadrant.
She shot back, ‘Quadrant’?
I said ‘yes. Do you know what is a quadrant’?
She shook her head and sheepishly said, ‘I’ve forgotten. You mean 270 degrees?’
I asked ‘What is the first quadrant’?
‘Zero’
Without revealing any anger in my voice, I asked, ‘What is the range of the first quadrant’?
‘Zero. No, 90’
‘What is the second quadrant’?
‘180’
‘What is the third quadrant’?
‘270’
At that point I lost my patience and told her, ‘First quadrant is from 0 to 90. Can you now identify the 3rd quadrant’?
‘Yes sir. It is 180 to 270’.
Quite relieved at this huge success, I said, ‘Can you now just calculate 16 samples of a full wave and show me the result’?
She came back after 15 minutes and showed me a set of calculations that were all wrong. She had no idea what she had to do.
I thought I would go to the absolute basics and asked her ‘What is sin 30’?
She quickly whipped out her scientific calculator. I said, ‘You don’t need a calculator for that. Can you not draw a triangle and calculate’?
She stared at me as if I was out of my mind. Then she drew a vague triangle in which not even one angle was a right angle.
So, I drew one and denoted x as the ‘opposite’ and y as the hypotenuse. I said ‘Can you now calculate sin 30’?
‘But both x and y are unknown’.
I helped her by saying that ‘y’, the hypotenuse was 1. ‘Can you now calculate x’?
She quickly and triumphantly wrote ‘x= y*sin 30’!
‘I think you can calculate the value of x in relation to y, can’t you’?
An empty stare.
‘If one angle is 30 in a right-angled triangle, what would be the other?’ I asked.
’30 degrees’!
‘What is the sum of all three angles in a triangle’?
‘180. So, the other angle should be 60’.
So, I drew a mirrored triangle beneath the existing one to create the resultant equilateral triangle and asked ‘Does this shape give you any hints?’
An empty stare. So, I asked ‘Do you see any symmetry in the super triangle’?
‘Yes! If one is x, the other is (1-x)’!
I slapped my forehead and said ‘If there’s an isosceles triangle, can you guess x’?
‘It is x/2’.
With many more minutes of prodding and slapping my forehead, she arrived at ‘sin 30 = 0.5’
‘Now that you’ve managed to calculate sin 30, can you now calculate sin 45′?
She drew another triangle just like the 30 degree triangle, wrote x=0.5 and y=1 and marked the angle as ’45’.
I remarked ‘How did you mark x as 0.5′?
‘Sir, we just worked it out’!
I let it be and asked, ‘If one angle is 45 in a right angled triangle, what is the other angle’?
I was quite relieved that she did not go for her calculator. She actually blurted out ’45’ in just under 35 secs.
‘Great! If two angles are 45, can you figure out any relationship between any two sides’?
‘The base (adjacent) will be root 2’.
I said ‘If the two angles are 45, which two sides would be equal’?
Losing patience, I identified the base and the opposite sides as ‘1’. ‘Can you now calculate the hypotenuse’?
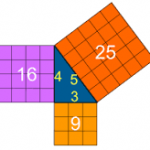
A blank stare forced me to draw dotted squares on the three sides and I asked ‘Does this picture now tell you anything’?
She shook her head. I asked ‘Have you heard of Pythagoras theorem’?
‘I have forgotten, Sir’.
Assuming that x, y & z may be more confusing than the a,b & c that we used to be taught in school, I wrote the latter.
No use.
So, I just wrote the formula c2 = a2 + b2.
Voila! ‘Root 2’ came the answer at last!!!!
‘Now that you have managed to calculate sin 30 and sin 45, can you now do sin 60’?
‘Sure’ was the very confident and proud reply.
She proceeded to draw yet another triangle that looked exactly like the first one and promptly wrote 60 in place of the 30.
She wrote ‘1’ on the hypotenuse and ‘1.5’ on the side opposite 60.
I was horrified.
‘How did you get 1.5 on that side’?
‘For a 15 degree increase from 30 to 45, that side increased from 0.5 to 1. So, for another 15 degree increase, it will increase by another 0.5’!
I thought to myself, “Absolutely brilliant logic”, but preferred to tell her calmly, ‘That’s not correct logic. If that was so, what would happen if the angle increased to 90’?
She proceeded to write two superimposed vertical lines for some distance and said, ‘It will be 2’.
‘How did you get 2? Why not 2.5’?
‘No, it can’t be 2.5’
‘But, you know what sin 90 is in reality, don’t you’?
‘Yes. 1’.
‘So, isn’t your logic wrong’?
‘Yes, Sir’.
‘So, now go back to your first triangle that you drew for sin 30. There’s something that you can see right there for 60’, I said.
She didn’t get it. So, I pointed out the 60 degree angle at the top of the triangle and asked ‘Can you write the sin 60 with reference to this angle’?
‘But that angle is in reverse. It goes beyond 180′.
I could not believe that I was listening to all this coming from an engineering graduate. Maintaining my composure, I took a deep breath.
I quickly drew another triangle as a mirror image of the 30 degree example and asked ‘Does this make any difference to the sin 30 just because the triangle is reversed’?
I was relieved when she said ‘No’.
‘So, can you now calculate sin 60 in the same triangle as the sin 30’?
‘Yes. It is 0.75’.
With anger and pain very visible on my face, I asked ‘How did you get that? Did you apply Pythagoras theorem’?
‘Oh yes. I forgot to do the squaring and rooting, Sir’!
If an engineering graduate has not understood the basics of what she studied in school, how did she not only progress through college but also get marks of over 60 and 70%? So, what’s the use of the examination system, not to talk of the class room lectures? If she does not even know the basic school-level geometry of a right angled triangle, let alone remember the name ‘Pythagoras theorem’, what science is she going to apply in life? What’s even more shocking to me is that many people tell me that 75% of the graduates are of this standard.
The question remains – If our engineering graduates do not learn how to apply basic mathematical, engineering and science concepts to solve a problem, what do we mean by “Make in India”?